We have learned that a simple, effective way to passively control steady-state vibrations is through "vibration isolation". As an example, consider a base-excited single-DOF system for which we would like to reduce its absolute response motion. For this case, the transfer function between the input base motion and the output displacement of the oscillator is the well-known transmissibility function. The characteristics of this transfer function are related to the frequency ratio r = omega/omega_n, where omega is the frequency of base motion and omega_n is the natural frequency of the system:
- For r < sqrt(2), the system displacement is amplified over the base motion.
- For r = sqrt(2), the system displacement is equal to the base motion, regardless of the damping in the system.
- For r > sqrt(2), the system displacement is reduced from the base motion. Increasing the damping in this frequency range actually increases the system displacement.
For effective vibration isolation, it is desirable to increase the frequency ratio r to a value much larger than sqrt(2) by either reducing the stiffness of the system or increasing its mass. This is demonstrated in the simulation results below. As seen, for r = 4, the absolute motion of the system mass is effectively zero.
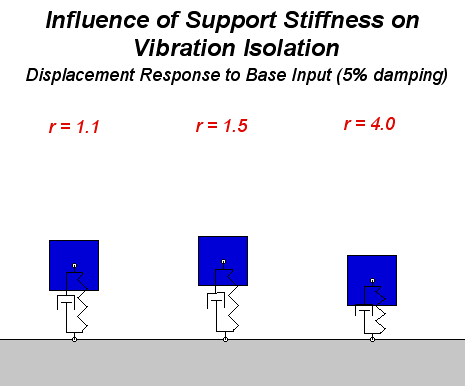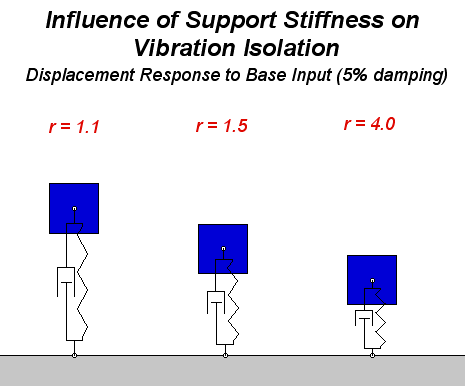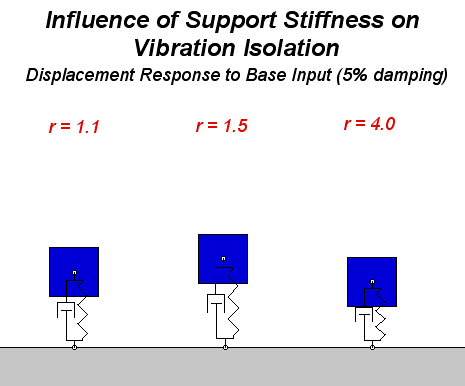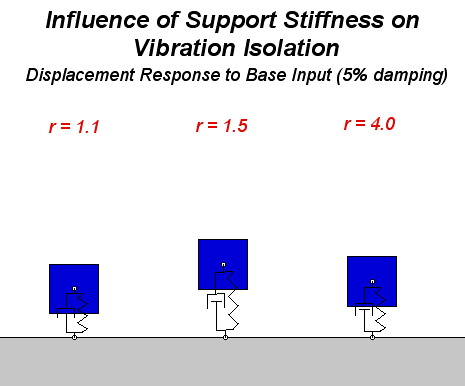 |
Also, as discussed above it is desired to keep the damping as small as reasonably possible for effective isolation. This is demonstrated in the simulation results below. As seen is this simulation, a 5% damping ratio in the isolation system is a very effective design, whereas the larger damping produces significantly larger amplitude response.
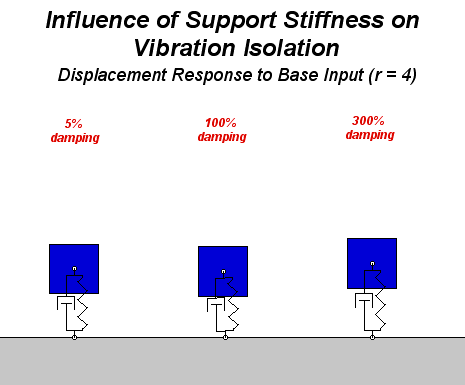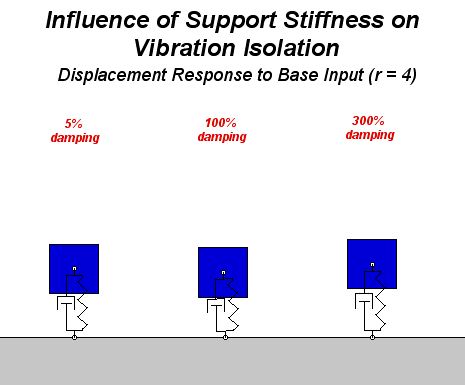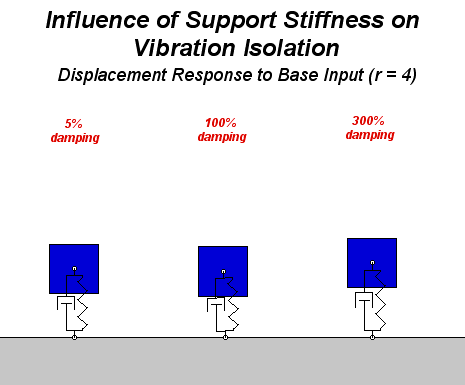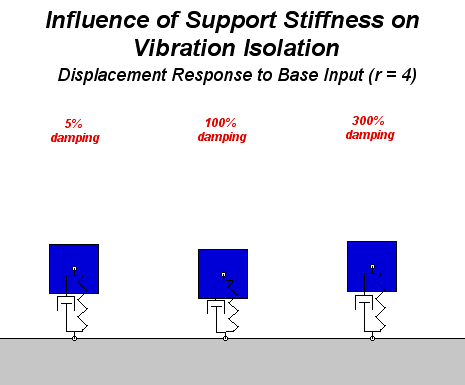 |