| Problem statement Solution video |
DISCUSSION THREAD
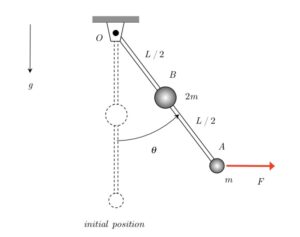
Discussion and hints:
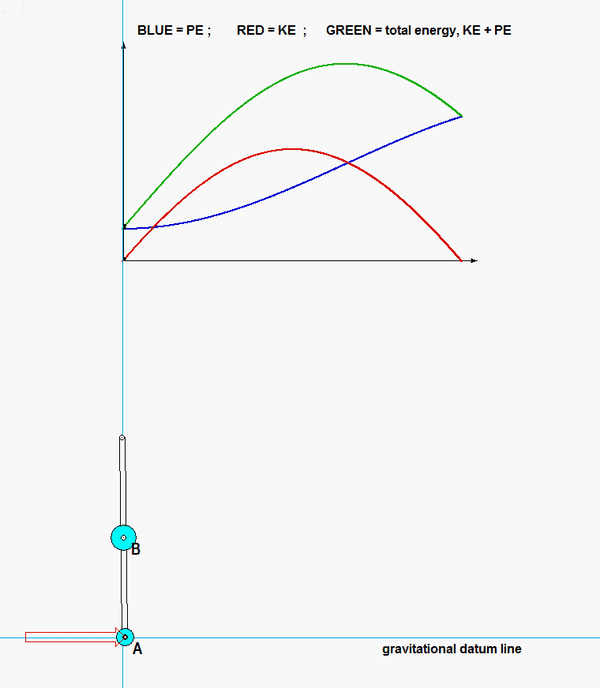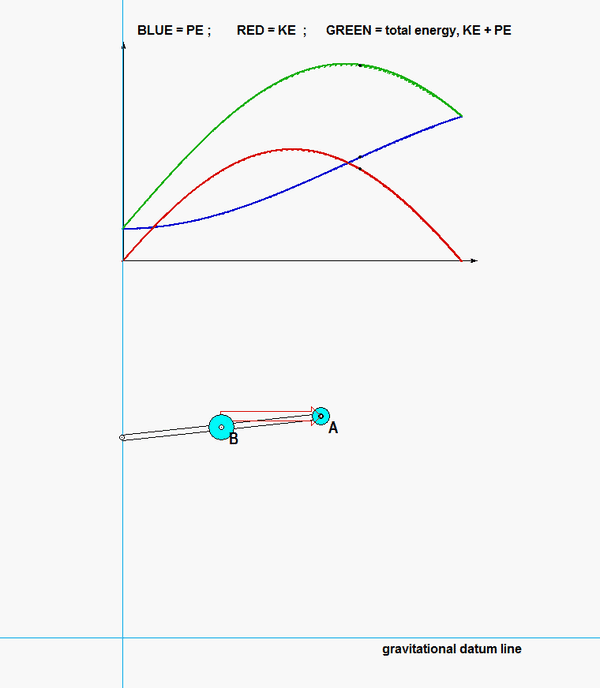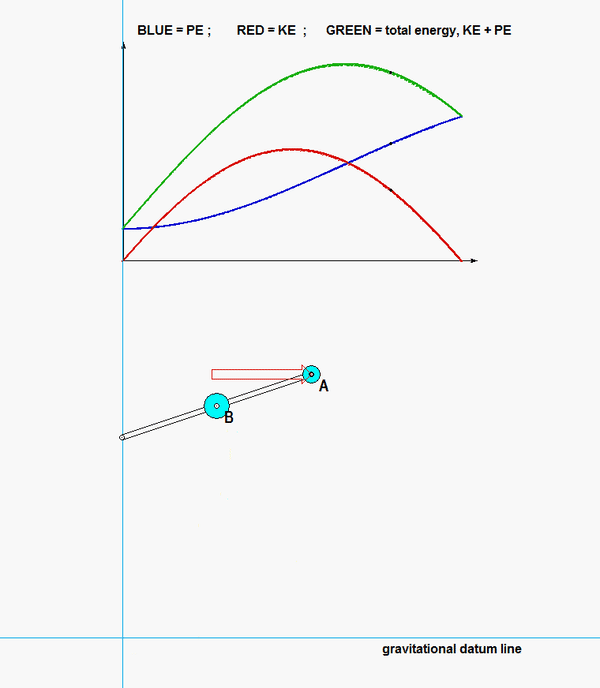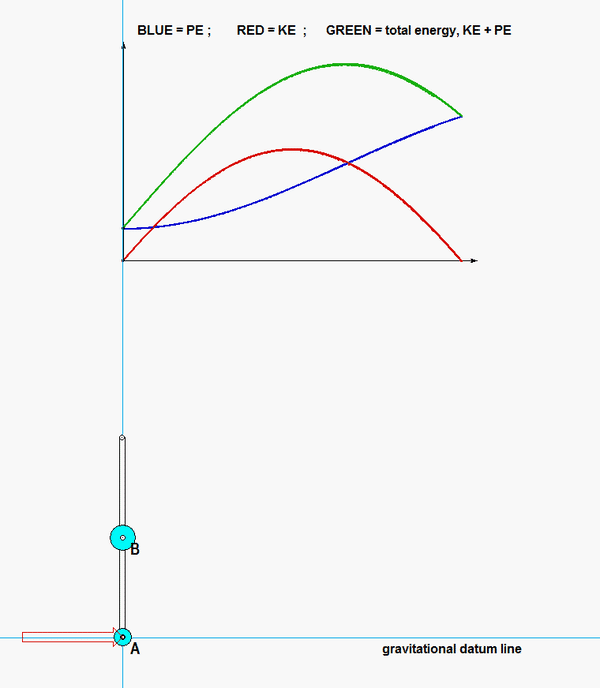
The constant applied force F acts only in the x-direction. Therefore, as explained in the lecture book, the work done by F is simply the force multiplied by the distance traveled by particle A in the x-direction. Use this result in the Step 2 of your analysis.
Recall the following four-step plan outline in the lecture book and discussed in lecture:
Step 1: FBD
Draw a free body diagram of the system made up of A+B+bar. Which, if any, forces do non-conservative work on this system? Can you justify this from the FBD?
Step 2: Kinetics (work/energy equation)
Write down the work energy equation for the system. The KE for the system is the sum of that for A and B. The PE for the system is the sum of that for A and B. Consider the above discussion when calculating the work done by the force F.
Step 3: Kinematics
What is the horizontal distance traveled by B? How are the speeds of A and B related to the angular speed of the bar?
Step 4: Solve
Solve your work/energy equation for the angular speed of the bar.
Any questions??
Are the only forces that do work here gravity and the applied force?
I had the same question, but I believe they both do work since A and B move in both the horizontal and vertical direction. However, I think you can consider gravity as part of the potential energy as well in the equation.
I believe those are the only two forces acting on the system. Gravity should go with the potential energy and then the applied force would go in the non-conservative work term.
Also, when solving for this problem it would be good to remember that the velocities at A and B are different when writing the kinetic energy part of the work energy equation.
Would we not be able to find the angular speed of the bar without finding the horizontal distance traveled? Are we required to find that first, then relate our findings to the angular speed in order to get the right answer?
You need to calculate the distance traveled by point A, but not the horizontal distance. The distance is measured along a path. I recommend using the arclength formula to find the distance traveled by A for a given angle of theta.
From what I'm seeing, the tension between the balls due to the rods shouldn't count as non-conservative right? because they're internal to the system?