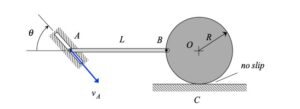
| Problem statement Solution video |
DISCUSSION THREAD
Ask and answer questions here. You learn both ways.
Discussion
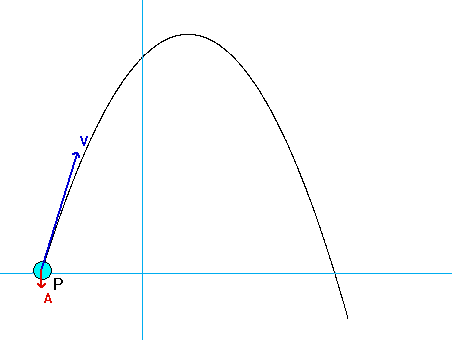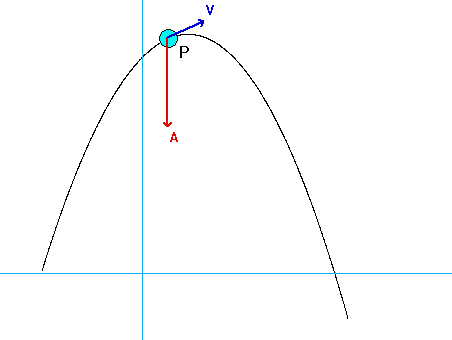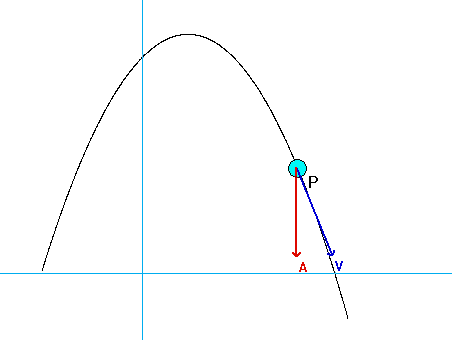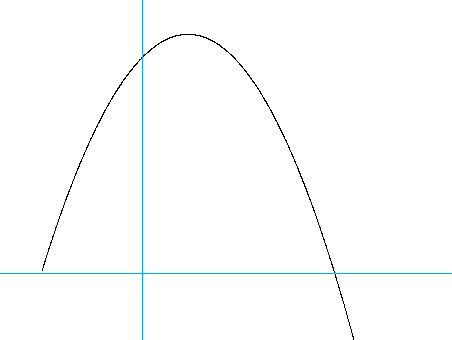
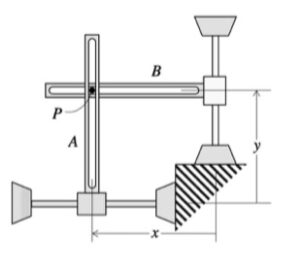
Consider the animation below of the motion for particle P:
- For all time, P moves with a positive x-component and a negative y-component of both velocity and acceleration. Why? Can you see this in the animation?
- As we will see in the next class period when we talk about the "path description" of kinematics, the velocity of a point is always tangent to the path of the point, and the acceleration always points "inward" on the path of the point. Do you see this in the animation?

The components of velocity and acceleration of P are found directly from the time derivatives of the position components:
vP = x_dot i + y_dot j
aP = x_ddot i + y_ddot j