| Problem statement Solution video |
DISCUSSION THREAD

Ask and answer questions here through Comments.
DISCUSSION and HINTS
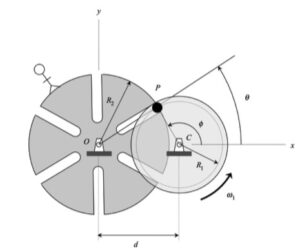
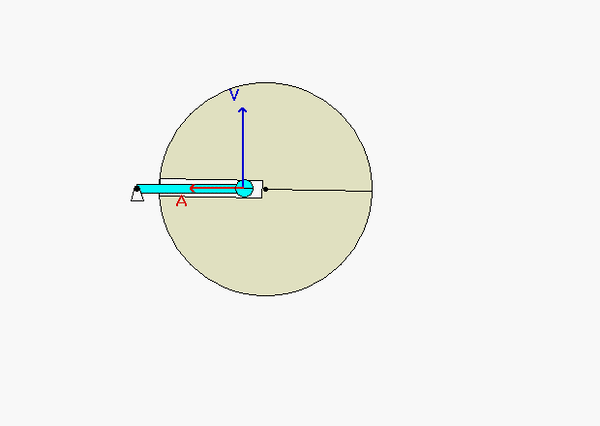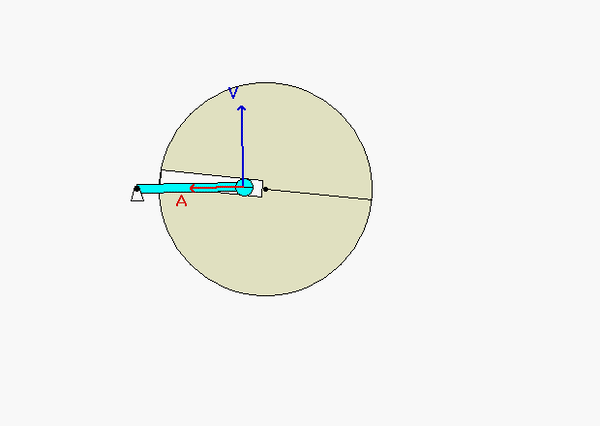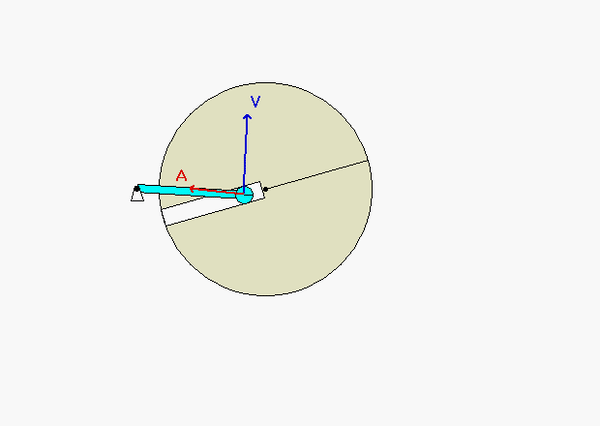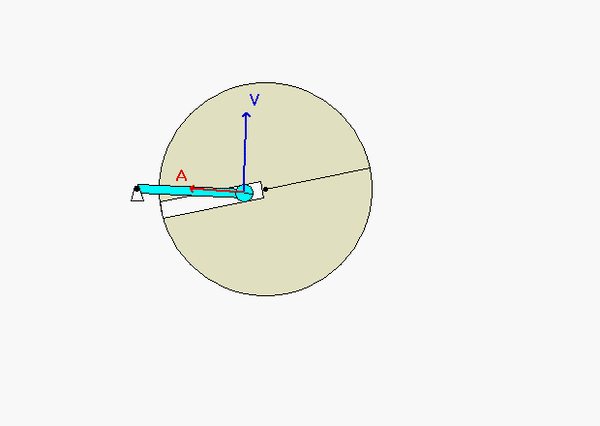
In this problem, we desire to relate the rotation rates of link AB and link OC. With the two rigid bodies connected by a pin-in-slot joint, we are not able to use the rigid body kinematics equations by themselves. Let's discuss that below.
Velocity analysis
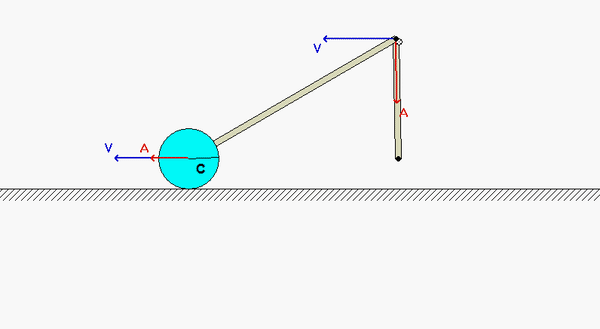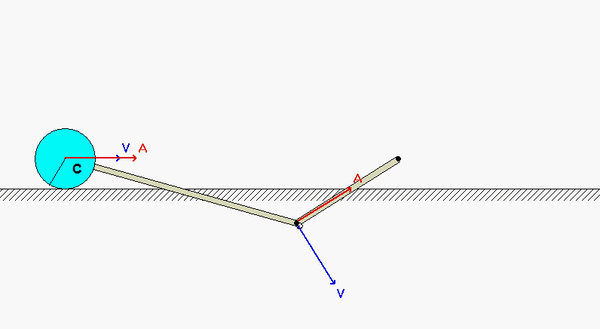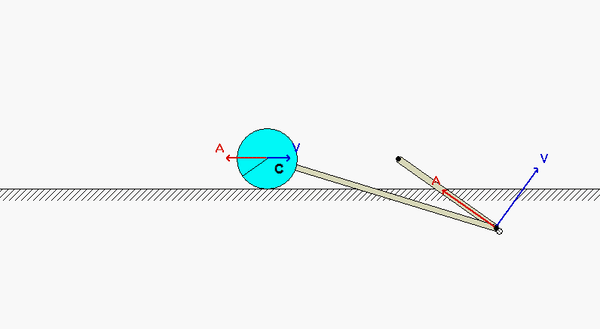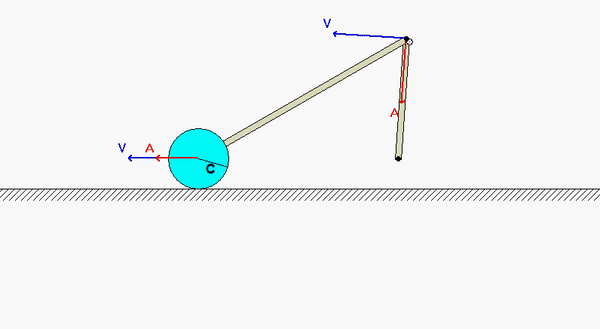
Here, we can use the rigid body velocity equation to relate the motions of A and B:
vA = vB + ωAB x rA/B
However, we cannot use a rigid body velocity equation to relate the motion of points O and A (the reason for this is that O and A are not connected by a rigid body). In its place, we can use the moving reference frame velocity equation with an observer attached to link OC:
vA = vO + (vA/O)rel + ω x rA/O
where ω is the angular velocity of the observer, and (vA/O)rel is the velocity of A as seen by our observer on link OC. Note that with the observer being attached to link OC, this observer sees motion of A only along the slot.
Combine these two equations to produce two scalar equations.
Acceleration analysis
We will use the same procedure for acceleration as we did for velocity - use a rigid body equation for AB and a moving reference frame equation relating O and A.
aA = aB + αAB x rA/B + ωAB x (ωAB x rA/B)
aA = aO + (aA/O)rel + α x rA/O + 2ω x (vA/O)rel + ω x (ω x rA/O)
where α is the angular acceleration of the observer, and (aA/O)rel is the acceleration of A as seen by our observer on link OC. Again, note that with the observer being attached to link OC, this observer sees motion of A only along the slot.
Combine these two equations to produce two scalar equations.