Ask and answer questions here. Think about helping out someone who has a question. You will learn as you help with a well thought-out answer.

DISCUSSION and HINTS
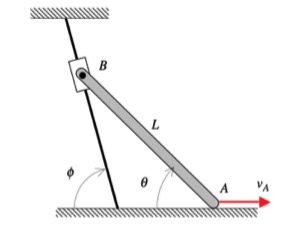
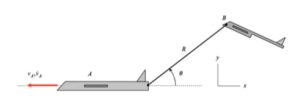
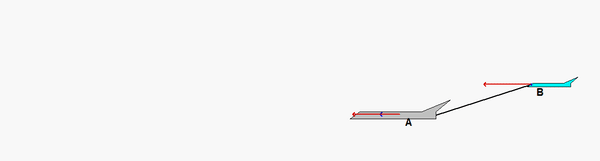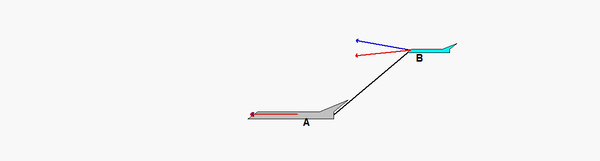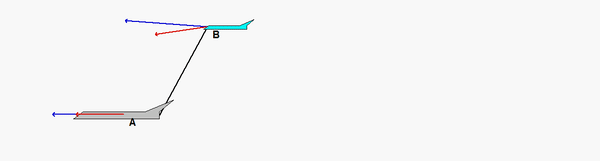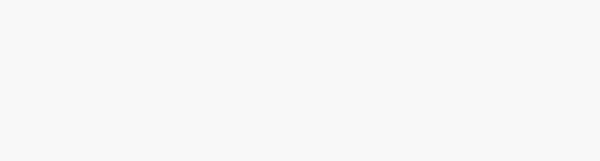
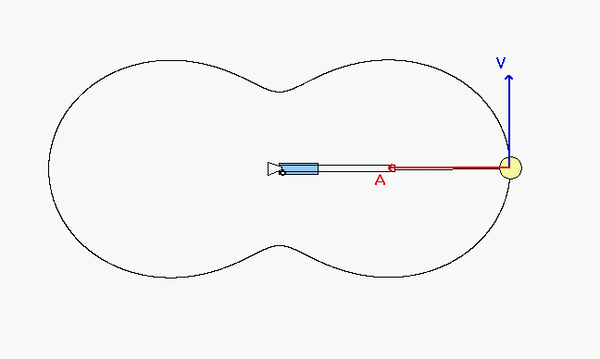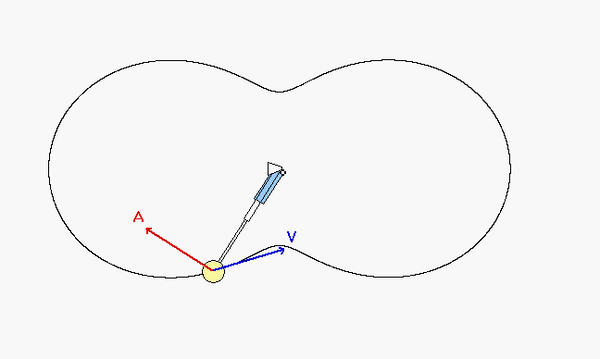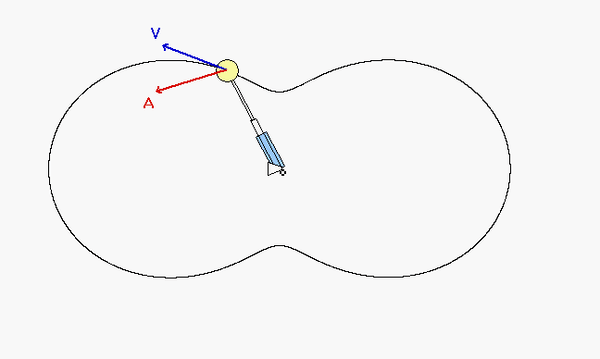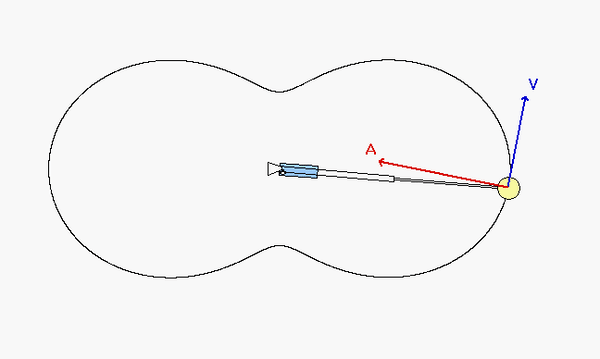
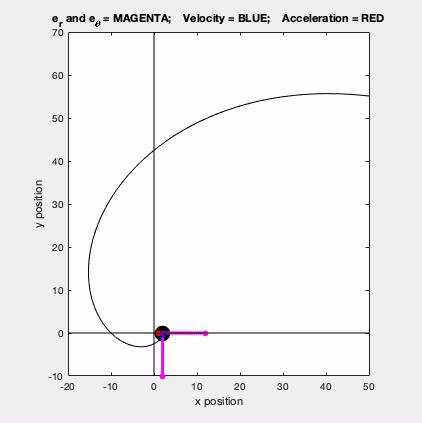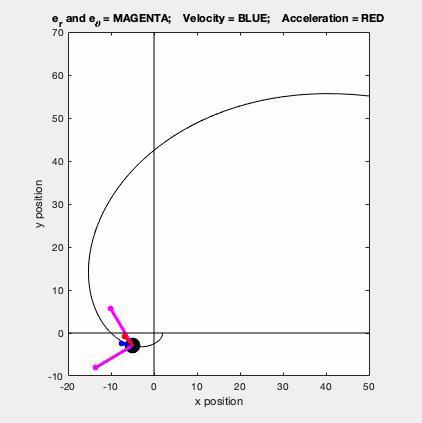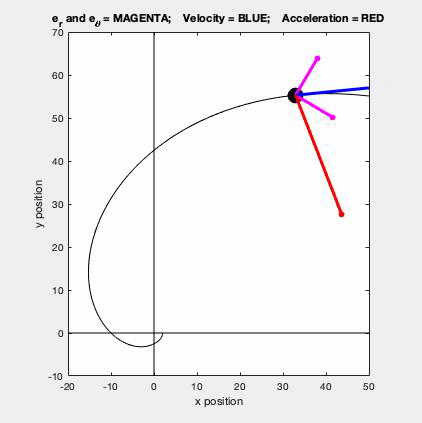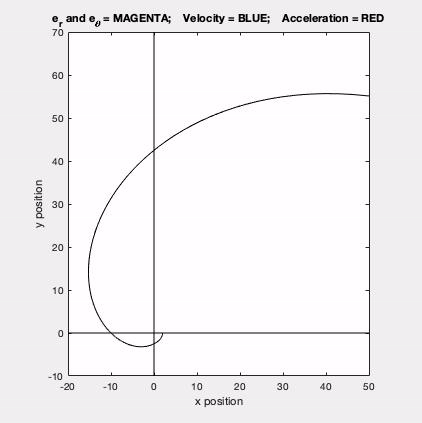
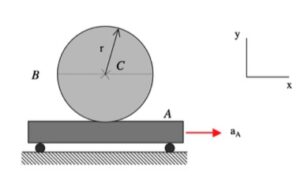
Block A moves to the right with a speed of v_A, with this speed changing at a rate of a_A. The disk rolls without slipping on the block in such a way that C has a known speed of v_C, with this speed changing at a rate of a_C.

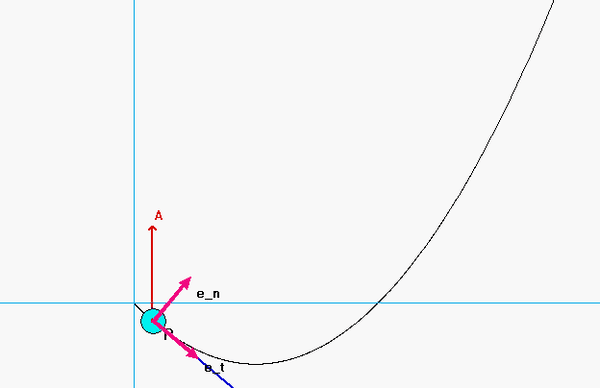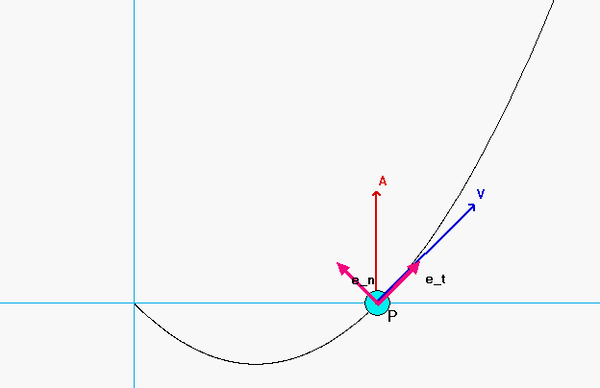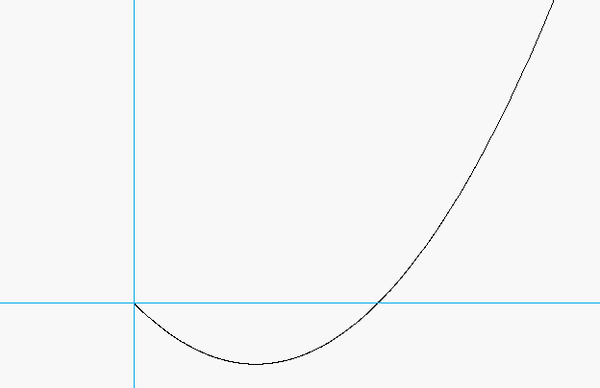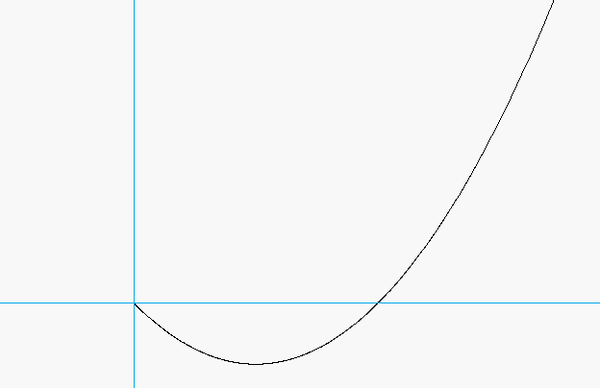
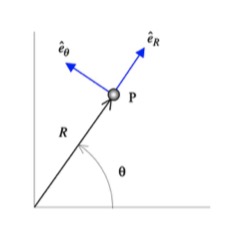
It is recommended that for this problem, you use the following rigid body kinematics equations relating the motion of the center of the disk C and the point on the disk that is in contact with block A (let's call that point E):

v_C = v_E + ω x r_C/E
a_C = a_E + α x r_C/E - ω²r_C/E

What do we know about the motion of point E? Point E has the same x-component of velocity and acceleration as does block A. The y-component of velocity of E is zero, but the y-component of acceleration of E is NOT zero. (Do you know why?)

Ask your questions here. And, consider answering questions of your colleagues here. Either way, you can learn.

DISCUSSION and HINTS

In this problem, end A of the bar is constrained to move along a straight horizontal path with a constant speed of v_A, whereas end B is constrained to move along a straight, angled path. As you can see in the animation below of the motion of the bar, the speed of B is NOT a constant (the acceleration of B is non-zero, and is, in fact, increasing as B moves along its path).

In your solution, it is recommended that you use the rigid body kinematics equations relating the motion of ends A and B:

v_B = v_A + ω x r_B/A
a_B = a_A + α x r_B/A - ω²r_B/A

For these equations, you know: i) the magnitude and direction for the velocity of A; ii) that the acceleration of A is zero (constant speed along a straight path); and, iii) the direction for the velocity and acceleration of B. These two vector equations produce four scalar equations that can be solved for four scalar unknowns: v_B, a_B, ω and α.

Ask and answer questions here. You can learn as you help others.

DISCUSSION and HINTS
The solution for this problem is a straight-forward application of our two rigid body kinematics equations relating the motion of point A back to point O:

v_A = v_O + ω x r_A/O
a_A = a_O + α x r_A/O - ω²r_A/O

where point O is fixed (zero velocity and zero acceleration).

Ask and answer questions here. Help out someone with a well thought-out answer to their question. You will learn as you explain.

DISCUSSION and HINTS

The motion of point C is quite complicated. However, its path is defined by the combination of motion from the translation of A with the rotation of the plate. As expected, the velocity of C is always tangent to its path.

The solution for this problem is a straight-forward application of our rigid body kinematics equations relating the motion of point C back to point A:

v_C = v_A + ω x r_C/A

where point A is translating to the right (positive x-direction) and the plate is rotating CCW at a rate of ω.

Ask or answer questions here. Try it. You will learn either way.

DISCUSSION and HINTS

Although end B of the cable is moving with a constant speed, block A is not. Can you see this in the animation below?

Write down the length L of the cable in terms of the two position variables s_A and s_B. Since the cable does not stretch, set dL/dt = 0. (Take care in performing the derivative dL/dt.) This will give you an equation that relates the speeds of blocks A and B.

Does your answer show that v_A < v_B (a result that is indicated in the above animation)? In fact, your result should show that 2v_A < v_B: does it?

Ask or answer questions here. You can learn from either.

DISCUSSION and HINTS
Since the cable does not stretch (R_dot = R_ddot = 0), an observer on aircraft A will see a circular path for B, although the true path of B is more complicated than that. Since θ_dot = constant, then θ_ddot = 0.

It is recommended that you write down the relative motion kinematics equations relating the motions of A and B:

v_B = v_A + v_B/A
a_B = a_A + a_B/A

and use a set of polar coordinates to describe v_B/A and a_B/A:

v_B/A = R_dot*e_R + R*theta_dot*e_θ = R*theta_dot*e_θ
a_B/A = (R_ddot - R*theta_dot^2)*e_R + (R*theta_ddot +2*R_dot*theta_dot)*e_θ = - R*theta_dot^2*e_R

You can ask questions or answer questions of others here. You can learn from either.

DISCUSSION
Shown in the animation below is the path taken by Particle P.

• As expected the unit vectors e_t and e_n are tangent and normal to the path of P, repectively.
• Also seen there is that the velocity vector is always aligned with the tangent unit vector, e_t, since v = v e_t.
• The acceleration vector, in general, has both tangential and normal components of acceleration. The normal component always points in the same direction as the unit normal vector, e_n. The acceleration vector either points "forward" of the motion (for which the rate of change of speed is positive; i.e., increasing is speed), or points "backward" of the motion (for which the rate of change of speed is negative; i.e., slowing down).

HINTS
Recall that since you do not know the y-component of motion of P explicitly in terms of time, you will need to use the chain rule to find dy/dt.

It is most convenient to determine the rate of change of speed through the vector projection of the acceleration vector, a, onto the unit tangent vector, e_t. How do you find the tangent unit vector? Simply divide the velocity vector by its magnitude!   e_t = v/v .

Once you know the rate of change of speed, you can find the radius of curvature ρ through the magnitude of the acceleration.

Ask your questions here. Or, if you can help someone by answering their questions, then do that here. You can learn either way.

HINTS
It is most convenient to determine the rate of change of speed through the vector projection of the acceleration vector, a, onto the unit tangent vector, e_t. How do you find the tangent unit vector? Simply divide the velocity vector by its magnitude!   e_t = v/v .

Once you know the rate of change of speed, you can find the radius of curvature ρ through the magnitude of the acceleration equation.

Do not confuse R with ρ - they are not the same thing.

Ask or answer questions here. Either way, you learn.

DISCUSSION and HINTS
The motion of P is shown in the animation below. As we saw in our last lecture, the velocity of a point is always tangent to the path of that point. The acceleration of a point will always have a normal component that points inward on the path. Can you make these general observations in the motion of P shown below? Can you tell from the animation when P is increasing in speed and when P is decreasing in speed? (Look at the direction of the tangential component of the acceleration relative to the direction of the velocity.)

Note that in this problem we are not given the radial variable r as an explicit function of time. Because of this, when we are finding dr/dt when need to use the chain rule of differentiation; that is, we should use: dr/dt = (dr/dθ)*(dθ/dt).

We encourage you to interact with your colleagues here on conversations about this homework problem.

With the polar description, we write the velocity and acceleration vectors for a point in terms of the polar unit vectors e_r and e_θ. These two unit vectors are shown in magenta in the animated GIF below.

• e_r points outward from the observer O toward point P
• e_θ is perpendicular to e_r and points in the positive θ direction.

The velocity (in blue) is seen to be tangent to the path, as expected, The  acceleration (in red) has both tangential and normal components: the normal component always points inward on the path, and the size and sign of the tangential component is directly tied to the rate of change of speed of P, also as expected.

In terms of problem solving, this problem is straight forward. Both r and θ are given as functions of time. The components of the velocity and acceleration vectors are found directly from the differentiation of r and theta with respect to time. The chain rule of differentiation is NOT needed here.

Some additional observations:

• Although r_ddot = constant, the r-component of acceleration is NOT constant.
• Although θ_ddot = 0, the θ-component of acceleration is NOT zero.