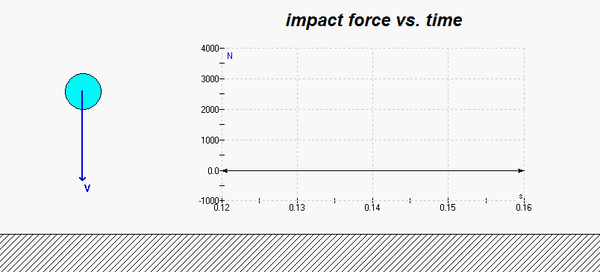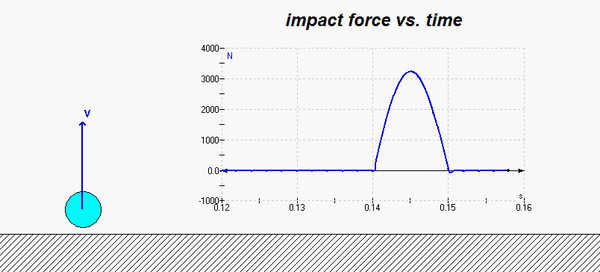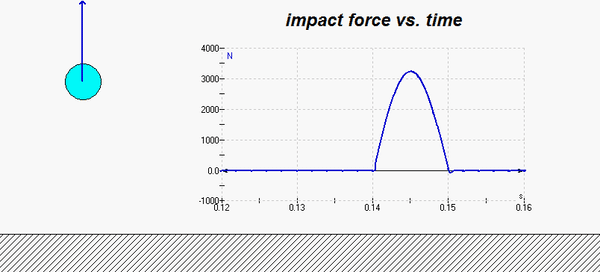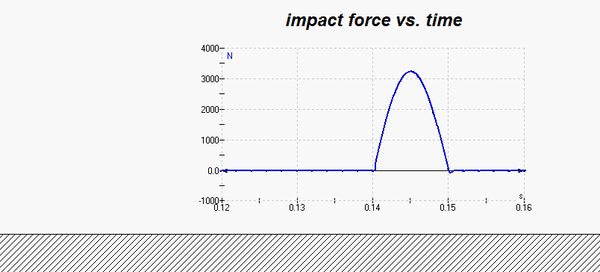
| Problem statement Solution video |
DISCUSSION THREAD
Discussion and hints:
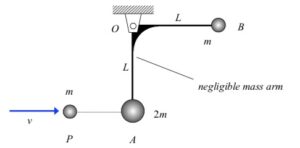
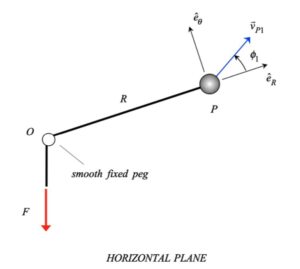
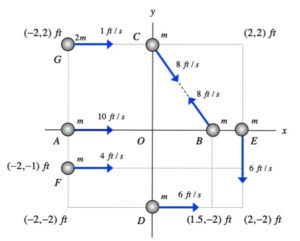
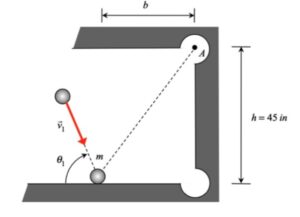
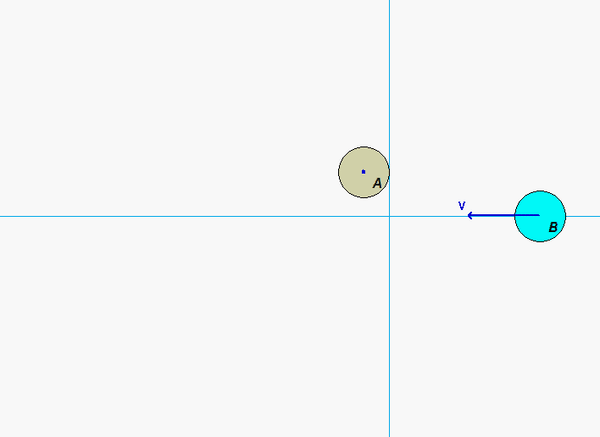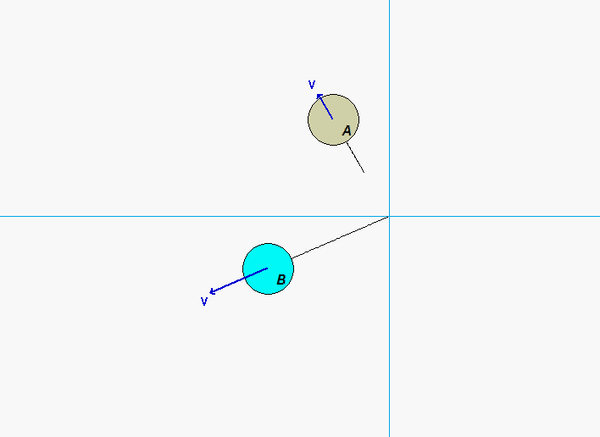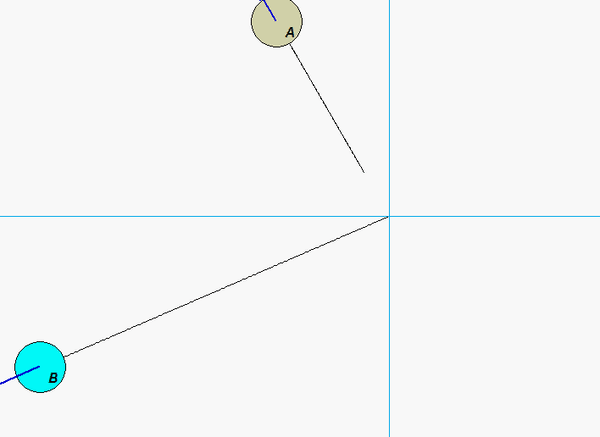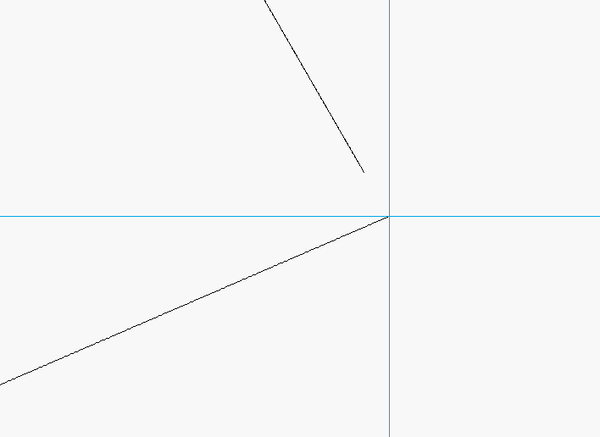
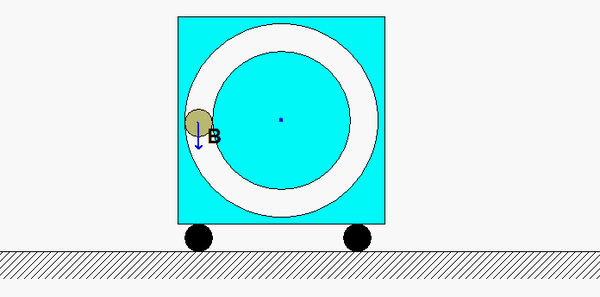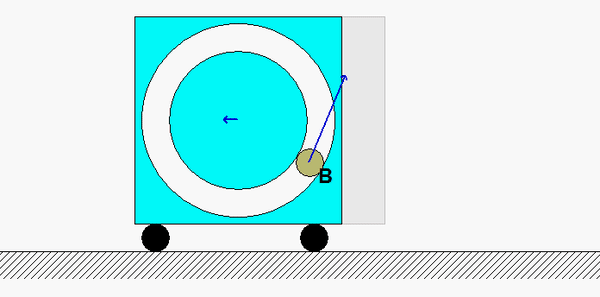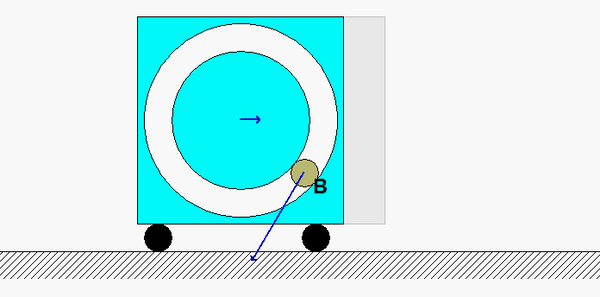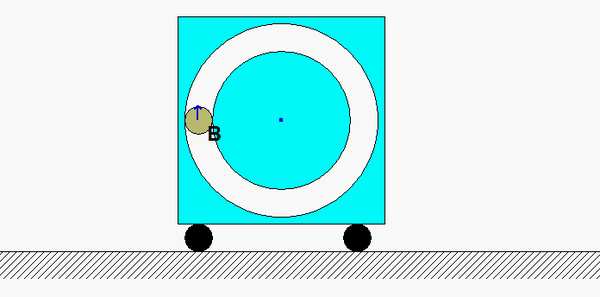
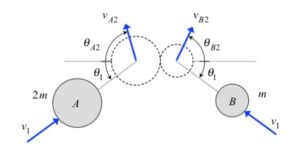
Recall that for oblique impact problems, it is recommended that you use three FBDs: A alone, B alone and A+B together. A+B together allows us to make the impact force internal (and not appear in the linear impulse-momentum equation). FBDs for A and B individually allows us to determine the t-direction components of velocity each for A and B.
Step 1: FBDs
Draw FBDs of A, B and A+B.
Step 2: Kinetics (linear impulse/momentum)
Consider using the linear impulse-momentum equation for the t-direction for A and B individually, and in the n-direction for A+B. You need four equations to determine the two components of velocity for each of the two particles. Consider the coefficient of restitution as your fourth equation.
Step 3: Kinematics
Step 4: Solve
Solve for the two components of velocity for each of the two particles. From these, the post-impact direction angles of motion can be found.
Any questions?