Discussion and hints:

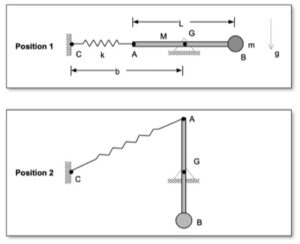
Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
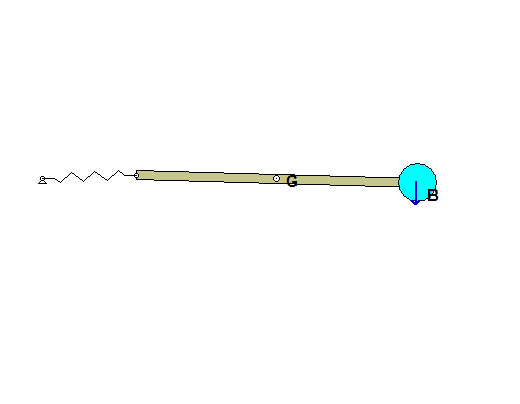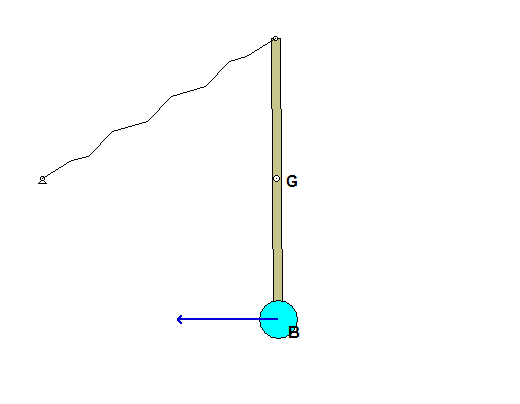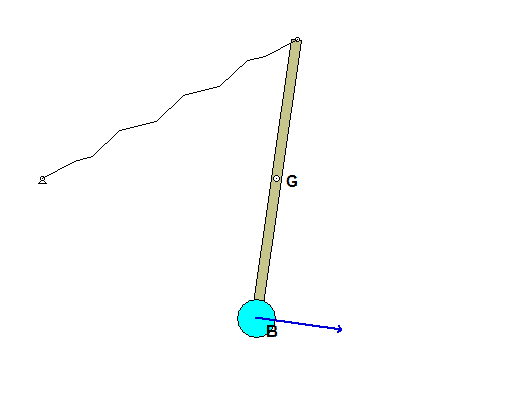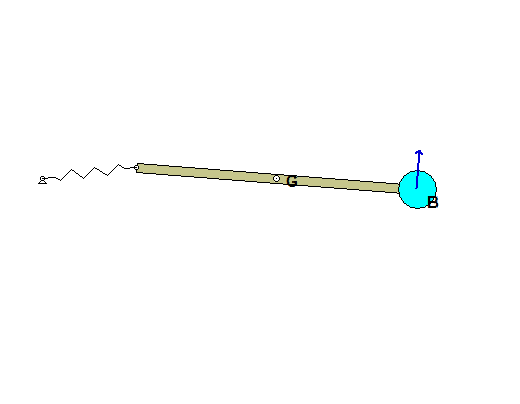
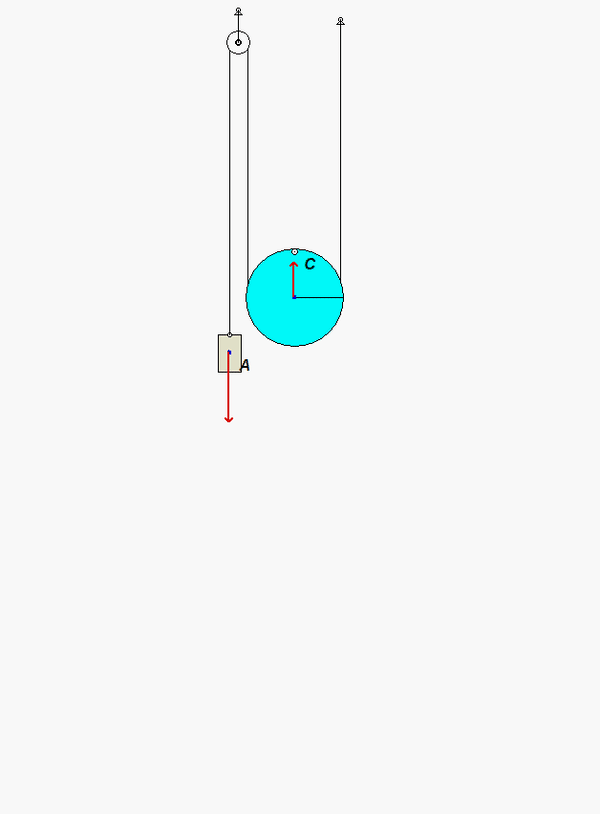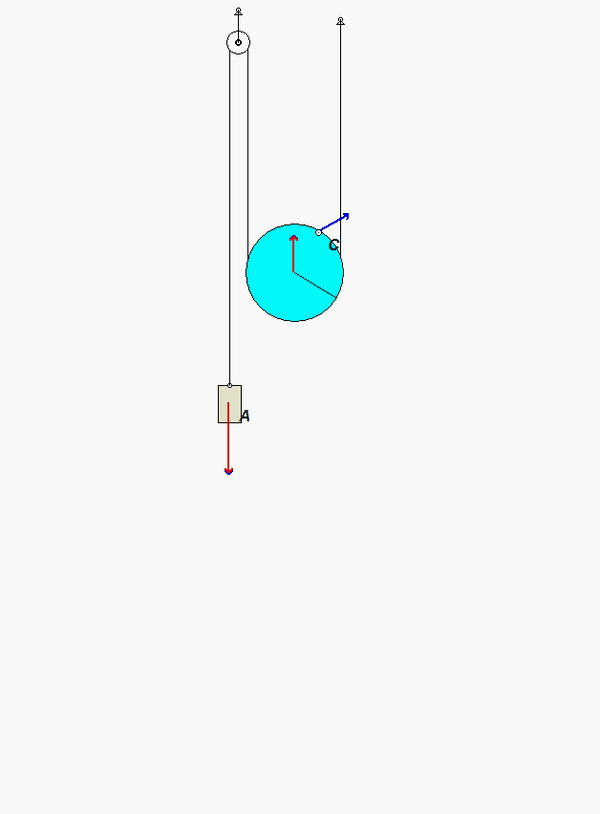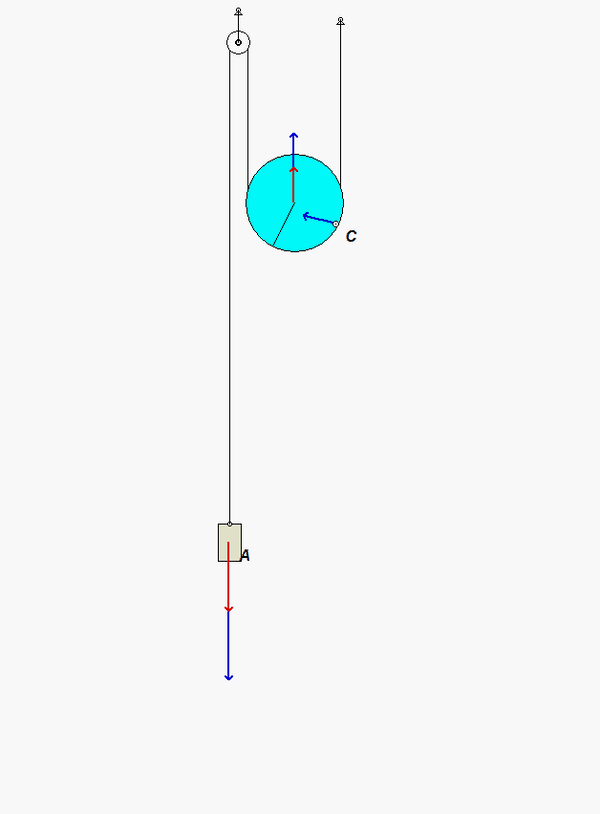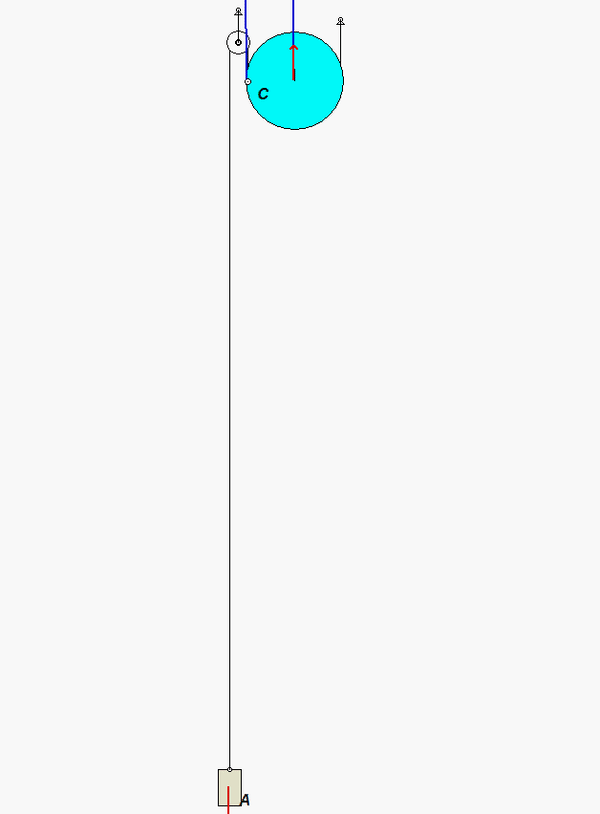
Draw individual free body diagrams for block A and the disk on the right. It is recommended that in drawing the FBD of the disk, include both the disk and the section of wrapped cable in the FBD, as shown below. This allows to you make the interaction forces between the cable and the disk "internal", greatly simplifying the FBDs. Also, please note that the cable tensions T_B and T_C are NOT the same.

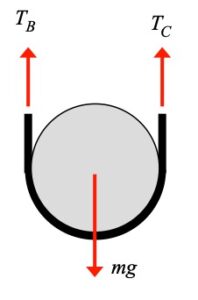
Step 2: Kinetics (Newton/Euler)
Write down the Newton/Euler equations for the block and the disk using your FBDs above. Take care in choosing the reference point for your moment equation for the disk. In order to use the "short form" of Euler's equation, this point should be either a fixed point, the body's center of mass, or a point whose acceleration is parallel to the vector connecting that point to the center of mass of the body. For this problem, there are no fixed points. However, the latter two options will apply. You might consider using point C since its acceleration will always point toward the center of mass of the disk. If you are not confident with this, then stick with the center of mass of the disk.

Step 3: Kinematics
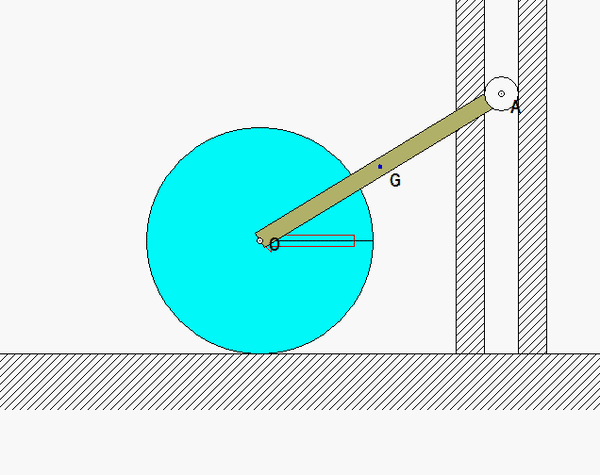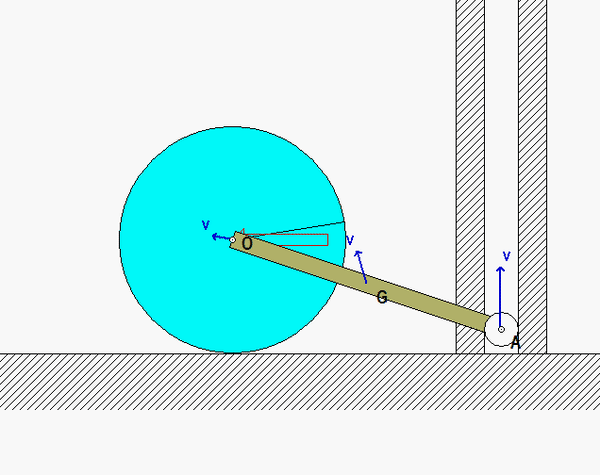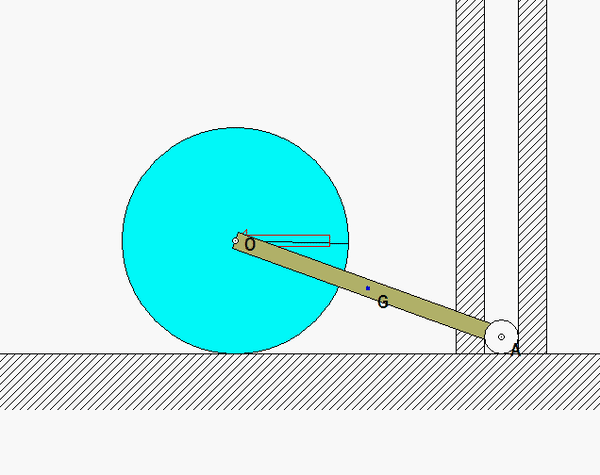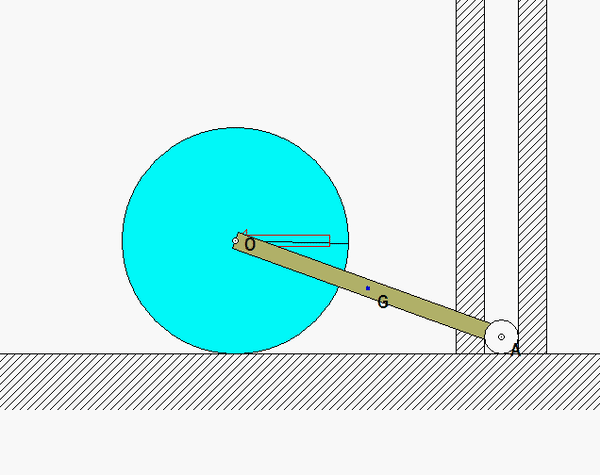
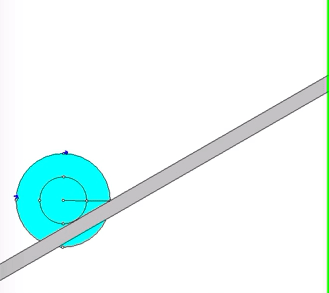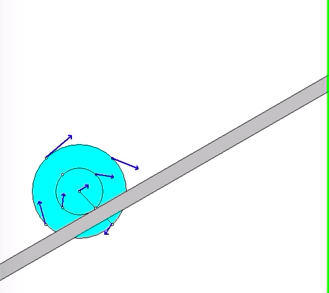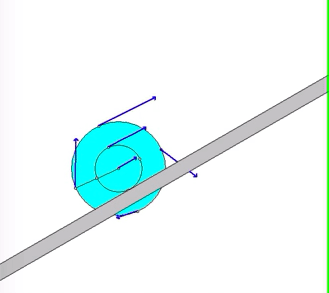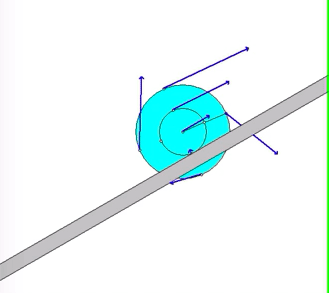
Put some thought into the kinematics for this problem. Note that the contact point on the disk with the cable on the right side (point C) is the instant center for the disk. (Recall that the cable does not slip on the disk. Since the cable on the right side is stationary, then so is point C on the disk.) This is apparent when you view the animation above of the system as it moves - as point C moves into contact with the cable, its velocity is zero. This will assist you in being able to relate the motions of the center of the disk to the motion of block A.

Recall that since C is the IC of the disk, it can have, at most, a component of acceleration in the x-direction. With this, you can use the kinematic analysis below to relate the acceleration of the center of mass of the disk to its angular acceleration, and to relate the accelerations of the disk center of mass of point B.

NOTE: The acceleration of A is NOT equal to the acceleration of the center of the disk. Ask questions here on the blog if this is not clear.

Step 4: Solve
Solve your equations above for the acceleration of block A.

Any questions?

Discussion and hints:

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a single free body diagram for the system made up of the disk and rod combined.

Step 2: Kinetics (work/energy)

• Write down the kinetic expression for the disk and rod individually, and then add those together to find the total KE for the system of your FBD. For each KE expression, recall that your reference point needs to be either the center of mass of the body, or a fixed point on the rigid body. You might consider using the no-slip, rolling contact point as your reference point for the disk.
• Do the same for the potential energies: write down the PEs for each body individually and add together.
• Also, based on your FBD above, which, if any force, does nonconservative work on the system in your FBD? Determine work for such a force.

Step 3: Kinematics
Note that the instant center (IC) for the disk is the no-slip contact point C. Where is the IC for rod OA at position 2? You might want to review Chapter 2 of the lecture book in finding the IC for a rigid body moving in a plane. (Carefully study either the animation above for position 2 or the freeze frame for that position below - you can actually see the IC from these!) Locating this IC is critical for you in setting up and using the kinematics for this problem.

Step 4: Solve
Solve your equations above for the velocity of point A.

Any questions?

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

The animation below shows the impact of particle B with the bar.

Freeze-frame of motion immediately after impact.

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a single free body diagram (FBD) of the particle and bar.

Step 2: Kinetics (impulse/momentum equations)
Using your FBD above, sum moments about point A . Consider the time of the impact to be short such that there is no change in position of either the bar or B during impact. Also, consider the particle to be of small physical dimensions.

What does your moment equation above say about the angular momentum of the system about point A?

Step 3: Kinematics
What kinematics do you need to solve this problem?

Step 4: Solve
From your equations in Steps 2 and 3, solve for the angular velocity of the bar immediately after impact.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

The animation below shows the impact of the particle with the rigid bar. As stated in the problem statement, the particle sticks to the bar during the short impact time.

Considering the system made up of the particle and the bar, we see that there are no fixed points that are easily recognized and determining the location of the center of the mass requires some calculation. Because of this, it is advisable to consider the particle and the bar in separate FBDs in your analysis.

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw individual free body diagrams (FBDs) of the particle and bar. Be sure to draw the impact force on both FBDs.

Step 2: Kinetics (impulse/momentum)
Consider the linear impulse/momentum equation for the particle and the angular and linear impulse/momentum equations for the bar. Note that each of these equations will include the impulse of the impact force.

Eliminate the impulse of the impact force from the above three equations. This will leave you with two equations in terms of the post-impact velocity of the particle, the post-impact velocity of the bar's center of mass, and the post-impact angular velocity of the bar.

Step 3: Kinematics
Since the particle sticks to the bar during impact, you can relate the post-impact velocities above through the rigid body kinematics equation:

v_B= v_G + ω_bar x r_B/G 

where B is the top point on the bar where the particle impacts and sticks.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the velocity of G and the angular velocity of the bar immediately after impact.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

The animation below shows the motion of the disk as it moves along the incline. Included in the video are the friction and normal forces (FF and FN) acting on the disk as it moves.

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a free body diagram (FBD) of the disk. In drawing your FBD, please note that the friction force is NOT proportional to the normal force N; that is, f ≠ μN. Why is that?

It is recommended that you choose a set of coordinates that are aligned with the ramp. For example, choose the x-direction down the incline and the y-direction   perpendicular to the ramp pointing up and to the right.

Step 2: Kinetics (Newton/Euler)

• Based on your FBD above, write down the impulse/momentum equation in the x-direction for the disk.
• Based on your FBD above, write down the angular impulse/momentum equation for the disk.
• Combine the two equations above by eliminating the impulse of the friction force from the equations.

The above gives you a single equation in terms of two variables: v_O and ω_disk.

Step 3: Kinematics
Note that the no-slip contact point of the disk with the incline is the instant center (IC) of the disk. Let's call that point C. Since C is the IC of the disk, you can readily relate the angular velocity of the disk to the velocity vector of the disk center O through:

v_O= v_C + ω_disk x r_O/C = ω_disk x r_O/C

Be careful with signs.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the velocity of point O on the wheel at time 2.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a free body diagram (FBD) of the disk.

Step 2: Kinetics (Newton/Euler)
Based on your FBD above, write down the linear impulse momentum and angular impulse/momentum equations for the disk.

Step 3: Kinematics
What kinematics do you need here to solve?

Step 4: Solve
From your equations in Steps 2 and 3, solve for angular velocity of the disk at time 2 and the reactions at O.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS
As the bar falls from its initial position, block B is moving downward. Once the bar has moved to a position where bar OA is aligned with section AC of the cable, the direction of motion for B is reversed and B begins moving upward. As can be seen in the animations, at some points in the subsequent motion, the cable goes in and out of being slack. What causes the cable to go slack?

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a single free body diagram (FBD) of the system made up of bar OA and block B.

Step 2: Kinetics (work/energy)

• Looking at your FBD above, which forces, if any, do work that is not a part of the potential energy of the system? If there are none, then energy is conserved.
• Write down the individual kinetic energy expressions for bar OA and block B, and add together for the total KE of the system.
• Define your gravitational datum line. Write down the individual potential energy expressions for the bar OA and block B, and add together for the total PE of the system.

Step 3: Kinematics
Relate the speeds of A and B, and relate the speed of A to the angular speed of bar OA. It is recommended that you use some polar coordinates with the origin at C. See the notes that follow. Note that since the cable does not stretch, the value of R_dot is the same as the speed of B.

A freeze-frame of the above animation is shown below for the position where OA is horizontal. Note that the speed of A is larger than the speed of B, as is indicated in the kinematic analysis above.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the velocity of block B at position 2.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a single free body diagram (FBD) of the system made up of the drum, block A and the spring.

Step 2: Kinetics (work/energy)

• Looking at your FBD above, which forces, if any, do work that is not a part of the potential energy of the system? Does the force of friction at the no-slip contact point do work? If there are none, then energy is conserved.
• Write down the individual kinetic energy expressions for the drum and block A, and add together for the total KE of the system.
• Define your gravitational datum line. Write down the individual potential energy expressions for the drum block A and the spring, and add together for the total PE of the system.

Step 3: Kinematics
Relate the speed of A to the angular speed of the drum.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the velocity of block A at position 2.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a single free body diagram (FBD) of the bar and the particle.

Step 2: Kinetics (Work/energy)

• Looking at your FBD above, which forces, if any, do work that is not a part of the potential energy of the system? If there are none, then energy is conserved.
• Write down the individual contributions to the kinetic energy from the bar and particle, and add together for the total kinetic energy of the system.
• Define your gravitational datum line. Write down the individual contributions to potential energy from the bar, particle and spring, and add together for the total potential energy.

Step 3: Kinematics
Use the following rigid body kinematics equation to relate the angular velocity of the bar to the velocity of the particle B at position 2:

v_B= v_G + ω_AB x r_B/G

Step 4: Solve
From your equations in Steps 2 and 3, solve for the angular velocity of the bar.

Ask your questions here. Or, answer questions of others here. Either way, you can learn.

DISCUSSION and HINTS

The animation below shows the motion of the wheel as it is being pulled up the incline. The animation shows the velocity of a number of points on the wheel. Think about the location of the instant center for the wheel as it rolls without slipping, and how this location affects the direction and magnitude of the velocities shown here.

Recall the following four-step plan outline in the lecture book and discussed in lecture:

Step 1: FBDs
Draw a free body diagram (FBD) of the wheel.

Step 2: Kinetics (Newton/Euler)

• Looking at your FBD above, which forces, if any, do work that is not a part of the potential energy of the system? Pay particular attention to the friction force at the no-slip point on the wheel - does it do work? How do you find the work done by the applied force F?
• Write down the kinetic energy of the wheel. Recall that the expression the KE for the planar motion of a rigid body is: T = 0.5*m*v_A² + 0.5*I_A*ω², where A is either the center of mass or a fixed point (fixed points include instant centers). So, in this case you can use either O or the no-slip contact point (let's call that point C).
• Define your gravitational datum line. Write down the potential energy for the wheel.

Step 3: Kinematics
Use the IC approach to relate the velocity of the point on the wheel where the cable comes off (call that point A) to the angular velocity of the wheel. Or, instead, use the following rigid body kinematics equation:

v_A= v_C + ω x r_A/C

Through a time integration of the relationship between the speed of A and the angular velocity of the wheel, you can determine the distance through which F moves.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the angular velocity of the wheel at position 2.