110 thoughts on “HOMEWORK 32 - Fall 24”

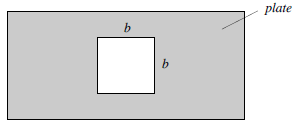
    2. The thickness of the plate is used to calculate the shear stress as shear stress is force applied over shear area. The shear area is the perimeter of the hole multiplied by the thickness t.

    3. The thickness allows us to calculate the 'inner wall' on which the shear stress is applied. Because it is a square, you would find the total perimeter of the square and multiply it by the thickness to find the area on which the shear stress acts.

      0

    4. Including the thickness t in the calculation is essential because it determines the total sheared area that resists the punching force. A thicker plate increases the sheared area, which, in turn, reduces the shear stress for a given force P.

    7. Shear stress occurs over the area that, in this case, is being sheared off. This is the perimeter of the rectangle that is cut off, so the thickness is needed to find the area of the rectangular prism that is actually removed.

    8. The thickness is essential in calculating the area of the perimeter over which the shear stress is applied. Thickness and the provided distance b are used to calculate this area.

    10. You need the thickness of the plate to calculate the surface area that is being cut. You’ll need the parameter of the square to calculate this as well, hope this helps.

    11. Thickness is required for finding the area. To find the area of the section being punched, you can use the perimeter multiplied by the thickness. Then you can solve for the shear stress.

    12. Thickness of the plate is considered when calculating the shear stress is because in this problem we are making a hole in the plate. If this problem only needed to make an indent in the plate, the thickness would be smaller because the shear would not go through the whole plate.

    15. The thickness helps calculate the inner wall where shear stress is applied. Since it’s a square, you find the total perimeter and multiply it by the thickness to get the area for the shear stress.

    2. I think that is because we are dealing with shear force which acts perpendicular to the surface, like if it were "cutting", so the force only acts on the perimeter. The thickness is eventually multiplied into the formula.

    3. Since the force doesn't act on the inside of the hole, the area is not b^2. Shear forces act on the perimeter of the sheet and through the thickness of the plate. Therefore, the area will be the perimeter multiplied by the thickness.

    4. Shear stress acts around the perimeter of the punch not the volume, so in this case you would be using the perimeter and later multiplying it by the thickness. This is because the punch only applies the force on the surface which later leads to the actual punching which is where thickness comes into play.

    6. Sheer acts on the perimeter because that is the surface that is being torn off in this scenario. If it was a whole solid bar and we are applying a torque/ force on it THEN will we use just the area.

    7. I think it’s because shear force acts perpendicular to the surface, like cutting, so it only applies to the perimeter. The thickness gets factored into the formula later.

    1. Punching force just refers to the fact the force is creating a hole by pressing a "punch", it is functionally the same as a regular force. However be aware of the units being ksi

    1. ksi is Kilopounds per square inch, and Pa is Newtons per square meter. Both are force divided by area, and the difference is only depending on what system you are using (metric or US customary)

  7. I think P is supposed to be in units of kips instead of ksi. To find the shear stress you divide P by the area giving you the shear stress to be in units of ksi.

    1. P can be in ksi, that just means you have to multiply it by the area it is acting on which is the square. Then when you solve, the square inches multiplied by ksi and the square inches in the denominator representing the area the shear stress acts on will cancel out leaving units of ksi.

      1

  8. Shear stress is in psi, which is pounds per square inch. Pa is just newtons per square meter, so Pa and ksi are used depending on the units presented in the problem

    1. If the punching force P is actually in kips (not ksi), then the answer for shear stress will indeed be in ksi after calculation. Since P should be in kips (thousands of pounds), you’ll calculate the shear stress by dividing P by the sheared area A (in square inches).

  10. To solve this problem, you first find the area of the hole punched by multiplying b^2 by t and then dividing P by that area. Are there more steps I am missing?

    1. To find the area where the shear stress occurs, you would have to use the perimeter of the punch than extend through t, rather than the normal area. Shear stress occurs on the edge of the shaft for this type of question.

    2. The perimeter of the square is what you need times the thickness for the area of the punch just like the lecture problem where it was a circular punch, circumference was used multiplied by the thickness. So it is P divided by the perimeter of the square times the thickness and that should end up in KSI.

    2. Towards the beginning of the semester, my professor said an FBD is always required on all homework even if it doesn't seem to be necessary. I'm sure it helps with keeping work organized and making things clear for the TAs.

      1

    3. I think it is still needed for overall visual clarity in the problem, you can draw the arrow for the punch force and also dimension add the needed dimensions including the thickness.

    4. I did a FBD of the area where the shear froce is applied expanded and showed P and the diretion of the shear, along with the dimensions. Im pretty sure the concpetual part of this problem they want us to show where the shear force is in the problem

  13. I am struggling to visualize how shear force acts "along the perimeter", can anyone explain? In the textbook, there is an example where the region being punched was circular, and the area that the shear force acted on was the circumference times the thickness. In this problem, would that translate to the area being the perimeter of the square times the thickness?

    1. Yes, it would be the perimeter times the thickness. When we are punching the hole, you can think of the area as the metal that gets exposed by punching out the hole. That is what had to be sheared through to punch the hole out.

    2. In this problem, the shear force acts along the perimeter of the square hole being punched. Since the hole is square with side length b, the shear area is calculated as the perimeter of the square (4b) times the thickness t. The shear stress is then found by dividing the punching force P by this shear area:
      τ= P/4b*t

    3. The shear force acts "along the perimeter" because the bonds between the molecules along that "plane" in the material are bonds that provide the reaction force against the force applied & break when there is material failure or over-stressing, causing the punched hole.

    4. The way I think of it is that the perimeter or edges of the square is what is "holding on" to the square that is attempting to be cut out of the metal sheet so that is where the shear force acts

    5. The way I think about it is the shear force acts along the perimeter because the molecular bonds along that plane provide the reaction force. These bonds break under overstress or material failure, causing the punched hole.

    1. The shear force is the force applied on the bonds between the molecules along that "plane" in the material (perimeter * thickness = area of stress that is parallel to the force applied) that provide the reaction force against the force applied & break when there is material failure or over-stressing, causing the punched hole.

    2. The shear force can be visualized as being spread evenly along the edges of the hole, where the punching force cuts through the material. For a square hole, it acts along the perimeter of the hole, which is 4b, multiplied by the plate's thickness t.

  18. I still included a FBD just to be clear, but I know it doesn't really seem necessary. Also I believe P was supposed to be in kips not ksi because ksi is the unit in the final answer.

  20. P should likely be in kips rather than ksi, since ksi is a unit of stress, not force. Using kips for P will result in shear stress in ksi after dividing by the area in square inches.

  22. This problem seems pretty straightforward, however one thing we need to note is that Shear stress is P/A, but A isn't exactly b^2 * t, it's actually perimeter * t, so 4b *t. This is just something I noticed that I was initially confused about.

    1. I was confused about that too. There is a difference between area and the shear area. Shear area is the perimeter, or in the case of a circular cut, the circumference times the thickness, whereas normal area is the basic area calculation.

    1. I believe that the final answer should be in kips/in^2 because the perimeter would be measured in inches squared while the force you can put it in kips so I would leave it like that.

  24. In determining the shear stress for the punching force, how does the thickness of the plate (t) influence the result? If we increased or decreased the thickness, how would that affect the necessary punching force to maintain the same shear stress? Additionally, would a different hole shape (like circular instead of square) change how we calculate shear stress, and if so, how?

    1. Well, for this problem the smaller the thickness the less punching force is required because there's an inverse relationship. I think it wouldn't change how you calculate the answer, just using different areas/parameters.

    2. As previously said, the units should be in ksi. As for the FBD, I would recommend drawing a top view FBD that clearly shows dimensions as well as an orthogonal one to show the vertical force components and thickness.

  26. Would the given punching force P to punch out the hole in the sheet create some sort of bending moment at the points where the sheet is held up? What about if the sheet is laying on a flat surface that has a hole in it directly below where the punched out section is. Would the bending moment(s) then be on the edge of the surface cutout?

  28. Is there a specific unit to use in the answer for this problem? When you do the calculations, the forces cancel out to equal kips, but I thought that was not a measure of stress.

  32. To solve this problem, I first calculated the area of the sheared surface by multiplying the perimeter of the square hole by the thickness of the plate, which is 0.1 inches. This gave me an area of 0.8 square inches. Then, I found the shear stress by dividing the punching force of 30 ksi by the area. The result was a shear stress of 37.5 ksi.

    1

Leave a Reply